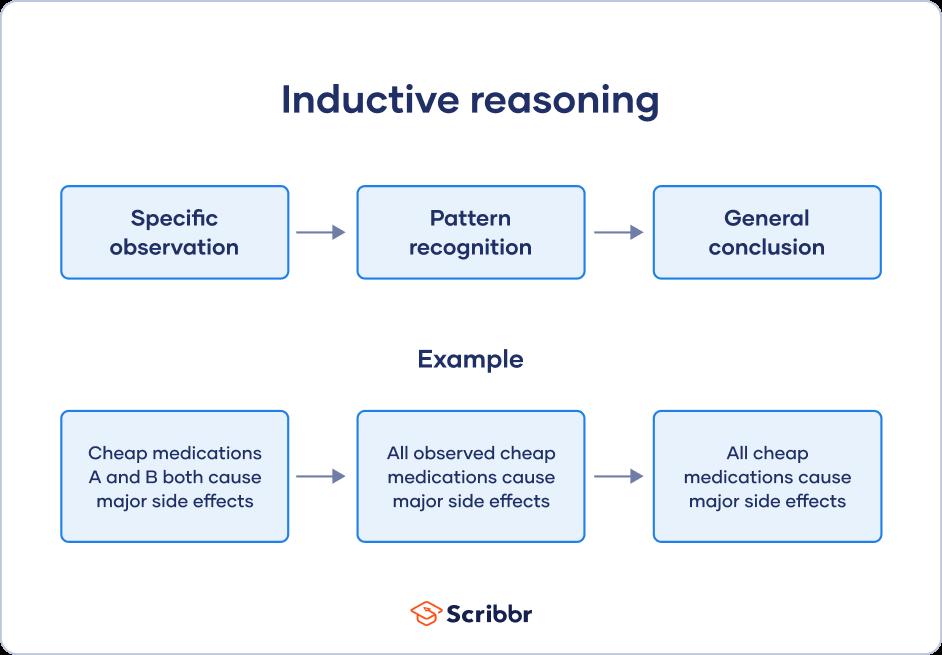
Definition
Forexample:inaplane,thesumoftheinternalanglesofarighttriangleis180degrees;thesumoftheinternalanglesofanacutetriangleis180degrees;thesumoftheinternalanglesofanobtusetriangleis180degrees;arighttriangle,anacutetriangleAndobtusetrianglesarealltriangles;therefore,thesumoftheinternalanglesofalltrianglesintheplaneis180degrees.
Inthisexample,fromtheindividualknowledgethattheinternalanglesofrighttriangles,acutetriangles,andobtusetrianglesare180degrees,respectively,thegeneralconclusionthat"thesumofalltriangleinternalanglesis180degrees"belongstoInductivereasoning.
Traditionally,inductivereasoningisdividedintocompleteinductivereasoningandincompleteinductivereasoningaccordingtothedifferentscopeofobjectsexaminedbythepremise.Completeinductivereasoningexaminesallobjectsofacertainclassofthings,whileincompleteinductivereasoningonlyexaminessomeoftheobjectsofcertainclassofthings.Andfurtheraccordingtowhetherthepremiserevealsthecausalconnectionbetweentheobjectanditsattributes,incompleteinductivereasoningisdividedintosimpleenumerationinductivereasoningandscientificinductivereasoning.
Moderninductivelogicmainlystudiesprobabilisticreasoningandstatisticalreasoning.Thepremiseofinductivereasoningisthenecessaryconditionofitsconclusion.
Secondly,thepremiseofinductivereasoningistrue,buttheconclusionmaynotbetrue,butmaybefalse.Ifarabbithitsatreeanddiedonacertainday,itcanbeconcludedthateverydayrabbitshitatreeanddie.Thisconclusionislikelytobefalse,unlesssomeveryspecialcircumstancesoccur,suchasanabnormalityinthegeographicalenvironment.Makestherabbitmusthitthetreeasfastaspossible.
Wecanusethestrengthofinductiontoillustratethedegreeofsupportofthepremisetotheconclusionininductivereasoning.Ifthesupportislessthan50%,thereasoningissaidtobeweakininduction;ifthesupportislessthan100%butgreaterthan50%,thereasoningissaidtobestrongininduction;ininductivereasoning,onlythepremiseofcompleteinductivereasoningsupportstheconclusionto100%,100%supportisinevitablesupport.
Thegeneralcalculationformofmathematicallogicofinductivereasoningis:s1⊆p+s2⊆p+s3⊆p+〈n〉(s⊆p)=∀×(s⊆p).
Comparisonofdeductivereasoning
Inductivereasoninganddeductivereasoningarebothdifferentandconnected.
Difference
1,thethinkingprocessisdifferent.
Thethinkingprocessofinductivereasoningisfromtheindividualtothegeneral,whilethethinkingprocessofdeductivereasoningisnotfromtheindividualtothegeneral,itisaninevitablethinkingprocess.
Deductivereasoningisnotfromtheindividualtothegeneralreasoning,butitisnotonlyfromthegeneraltotheindividualreasoning:deductivereasoningcanbefromthegeneraltothegeneral,suchas"Allunjustwarsareunpopular"Introduce"Allunjustwarsarenotpopular";
Itcanbefromindividualtoindividual,forexample,from"RogierBaconisnottheBaconwhoestablishedanewinductivelogictheory"tointroduce"TheestablishmentofanewinductionBaconinlogicaltheoryisnotRogerBacon";itcanbefromindividualandgeneraltoindividual,suchasfrom"thisobjectisnotconductive"and"allmetalsareconductive"todeducedthat"thisobjectisnotmetal";itcanalsobefromindividualandgeneraltoIngeneral,forexample,from"youarecompetentforthisjob"and"wherethereisawill,youcandoitorifyouarenotcapableofthisjob",theintroductionof"wherethereisawill,youcandoit."Here,weshouldpayspecialattentiontothefactthatthethoughtprocessofcompleteinductivereasoningininductivereasoningisnotonlyfromindividualtogeneral,butalsoinevitable.
2,therequirementsfortheauthenticityofthepremisearedifferent.
Inductivereasoningrequiresmajorpremises,andminorpremisesmustbetrue.Deductivereasoningdoesnothavethisrequirement.
3,thescopeofknowledgedeterminedbytheconclusionisdifferent.Theconclusionofdeductivereasoningdoesnotexceedthescopeofknowledgedeterminedbythepremise.Inductivereasoningexceptforcompleteinductivereasoning,theconclusionsarebeyondthescopeofknowledgedeterminedbythepremise.
4,thedegreeofconnectionbetweenthepremiseandtheconclusionisdifferent.Theconnectionbetweenthepremiseandtheconclusionofdeductivereasoningisinevitable,thatistosay,ifthepremiseistrueandthereasoningformiscorrect,theconclusionmustbetrue.Inductivereasoning,exceptthattheconnectionbetweenthepremiseandtheconclusionofthecompleteinductivereasoningisinevitable,theconnectionbetweenthepremiseandtheconclusionisprobable.
Contact
1,ifdeductivereasoningisbasedongeneralknowledge,(deductivereasoningdoesnotnecessarilyhavetobebasedongeneralknowledge),itusuallydependsoninductivereasoningToprovidegeneralknowledge.
2,Inductivereasoningisinseparablefromdeductivereasoning.First,inordertoimprovethereliabilityofinductivereasoning,itisnecessarytouseexistingtheoreticalknowledgetoanalyzetheindividualpremisesofinductivereasoningandgraspthecausalityandinevitability.Thisrequiresdeductivereasoning.Second,inductivereasoningreliesondeductivereasoningtoverifyitsownconclusions.Forexample,theRussianchemistMendeleevdiscoveredtheperiodiclawofelementsthroughinduction,andpointedoutthatthepropertiesofelementschangeperiodicallywiththeincreaseoftheatomicweightoftheelements.Later,itwasdiscoveredbydeductivereasoningthattheatomicweightsofsomeelementsthatwereoriginallymeasuredwerewrong.Soherearrangedtheirpositionsintheperiodictableandpredictedsomeundiscoveredelements,andpointedoutthatblankspacesintheperiodictableshouldbeleftfornewelementsthathavenotbeendiscovered.
Therehavebeentwoopposingfactionsinthehistoryoflogic-thefullinductivefactionandthealldeductivefaction.Theall-inductiveschoolregardsinductionastheonlyscientificwayofthinkinganddeniestheroleofdeductionincognition.Thefulldeductionschoolsaidthatdeductionistheonlyscientificwayofthinking,denyingthemeaningofinduction.Bothoftheseviewsareone-sided.AsEngelssaid:"Inductionanddeduction,justlikeanalysisandsynthesis,areinevitablyrelatedtoeachother.Oneshouldnotbesacrificedandtheothershouldbelifteduptothesky.Eachshouldbeusedwhereitshouldbeused,butToachievethis,wecanonlypayattentiontotheirmutualconnectionandtheirmutualcomplement."
Arrangementmethod
Experiencematerialsobtainedthroughobservation,experimentationandothermethodsneedtobeprocessedandarranged,Inordertoformascientificconclusion.Themethodsoforganizingempiricalmaterialsincludecomparison,classification,analysisandsynthesis,andabstractionandgeneralization.
Comparison
Comparisonisamethodtodeterminethecommonanddifferentpointsofobjects.Throughcomparison,boththesimilaritiesbetweenobjectscanbeunderstood,andthedifferencesbetweenobjectscanbeunderstood,soastoprovideabasisforfurtherscientificclassification.Usingthemethodofcomparison,itisimportanttorecognize"sameness"fromobjectsthatareextremelydifferentonthesurface,ortorecognize"differentness"fromobjectsthatarethesameorsimilaronthesurface.AsHegelsaid:"Ifapersoncanseetheobviousdifferenceatpresent,forexample,candistinguishapenfromacamel,wewouldnotsaythatthispersonhasgreatintelligence.Similarly,ontheotherhand,apersonCancomparetwosimilarthings,suchasoakandlocusttree,ortempleandchurch,andknowingthattheyaresimilar,wecannotsaythathehasahighabilitytocompare.WhatwerequireistobeabletoseethesimilaritiesanddifferencesDifferencesinthesame."
Whenmakingcomparisons,youmustpayattentiontothefollowingtwopoints:
(1)Compareunderthesamerelationship.Inotherwords,theobjectsarecomparable.Ifyoutrytomakecomparisonswithincomparablethings,youwillmakethemistakeof"comparisonwith".Forexample,thelengthofwoodisthelengthofspace,andthelengthofnightisthelengthoftime.Thetwocannotbeshorterthanthelength.
(2)Selectandformulateaccurateandstablecomparisonstandards.Forexample,biologicalspecimensarewidelyusedinbiology,andmineralspecimensarewidelyusedingeology.Theyareusedtoidentifydifferentspeciesoforganismsandminerals.Thesespecimensarethestandardforcomparison.Thestudyofmeteoritesorlunarrockmaterialscollectedbylandingonthemoonalsocomparesthemwithorespecimensontheearth.
(3)Compareinthesubstanceoftheobjects.Forexample,tocomparetwocollegestudentswhoarebetter,youmustcomparetheirideologicalandmoralcharacter,academicperformance,practicalabilityandothersubstantiveaspects,notgender,nativeplace,familyrichorpoor,etc.
Categorization
Categorizationisamethodofdistinguishingobjectsbycategorybasedontheircommonanddifferentpoints.Throughclassification,chaoticphenomenacanbeorganized,andalargenumberoffactualmaterialscanbesystematized.Classificationisbasedoncomparison.Throughcomparison,findoutthesimilaritiesanddifferencesbetweenthings,andthenclassifythefactualmaterialswiththesamepointsintothesamecategory,anddividethefactualmaterialswiththedifferencesintodifferentcategories.Forexample,thereareabout400,000speciesofplantsintheworld,whichcanbeclassifiedintofourcategories(phyla):thephylumAlgae,bryophyta,pteridophyteandseedplant.Fromthedoortothenext,youcangettheoutline,order,family,genus,andspeciesofunitsatalllevels.
Thereisadifferencebetweentheclassificationandthedivisionofterms.
(1)Thedirectionofthinkingprocessisdifferent.Lexicalitemsaredividedintosmallercategoriesfromlargercategories.Theclassificationistheopposite.Itstartsfromtheindividual,risestothecategory,andthenrisestothemoregeneralcategory.
(2)Differentroles.Thedivisionoflexicalitemsistoclarifythelexicalitems.Classificationisamethodofsystematizingthematerialpossessed.Moreimportantly,becausethecorrectclassificationsystemreflectstherelationshipbetweentheessentialcharacteristicsofthingsandtheinternalregularity,ithasscientificforesightandcanguidepeopletofindorrecognizenewconcretethings.Forexample,thenaturalclassificationsystemoforganismsestablishedonthebasisofDarwin'stheoryofbiologicalevolutionpredictedmanytransitionalorganismsthathadnotyetbeendiscoveredatthattime.ArchaeopteryxisthekindthatDarwinpredictedandfound.Archaeopteryxisanintermediatetypebetweenreptilesandbirds.Itfillsthegapbetweenthesetwotypesofanimals,indicatingthatbirdsevolvedfromreptiles.
AnalysisandSynthesis
Analysisisto"decomposethingsintosimpleelements".Synthesisis"combination,combination,andimprovisationtogether".Inotherwords,thethingsaredecomposedintocomponentsandelements,andaftertheresearchisclear,theycanbeputtogethertoshowthethingsinanewimage.Thisisthemethodofanalysisandsynthesis.
Forexample,toanalyzethestructureofanEnglisharticle,firstgetsentences,words,andfinallyget26letters;conversely,synthesisiscomposedofletterstoformwords,sentences,andthensentencestoformarticles.Thesearegrammars.Thesubjectmattertobestudied.Foranotherexample,thewhitelightpassesthroughtheprismanddecomposesintosevencolorsofred,orange,yellow,green,blue,andpurple;conversely,thesevencolorsoflightaresynthesizedintowhitelight.Thisistheanalysisandsynthesisofthespectrum,whichcanexplainthecauseoftherainbow.
Analysisandsynthesisaretwodifferentmethods,andtheyareoppositeinthedirectionofunderstanding.Buttheyarecloselyintegratedandcomplementeachother.Ontheonehand,analysisisthebasisofsynthesis;ontheotherhand,analysisalsoreliesonsynthesis.Withoutacertainsynthesisasaguide,itisimpossibletomakein-depthanalysisofthings.
AbstractionandGeneralization
Abstractionistheuseofthinkingabilityinpeople’sresearchactivitiestoexcludethesecondaryandnon-essentialfactorsoftheobject,andextractthemainandessentialfactors.Awaytoreachtheessenceoftheobject.
Summarizationisamethodofextendingtheunderstandingofthenatureandregularityoftheobjecttoallotherthingsofthesamekindinthinking.Forexample,afterdiscoveringthecommonnatureofthe"metal"that"canconductelectricity,"thiscommonnaturecanbeextendedtoallmetals,anditcanbesummarizedthatallmetalshavetheessentialpropertyof"conductingelectricity".
Completeinductionmethod
Concept
Completeinductionreasoningisbasedonthefactthateachobjectofacertaintypeofthinghasacertainattribute,therebydeducingthistypeofthingAllhavetheconclusionofthiskindofattribute.
Example
Forexample:"ItisknownthattherearemineraldepositsinEurope,mineraldepositsinAsia,mineraldepositsinAfrica,mineraldepositsinNorthAmerica,mineraldepositsinSouthAmerica,andmineraldepositsinOceania.TherearemineraldepositsinAntarctica,whileEurope,Asia,Africa,NorthAmerica,SouthAmerica,Oceania,andAntarcticaareallcontinentsontheearth.Therefore,allcontinentsontheearthhavemineraldeposits."Thelogicalformisasfollows:
S1isP
S2isP
……
SnisP
S1,S2,...,SnisoftypeSAllobjects
So,allSareP
Thecharacteristicofcompleteinductivereasoningisthatallobjectsofaclassofthingsareexaminedinthepremise,andtheconclusiondoesnotexceedtheknowledgedeterminedbythepremiseScope,therefore,theconnectionbetweenitspremisesandconclusionsisinevitable.
Toobtaincorrectconclusionsusingcompleteinductivereasoning,tworequirementsmustbemet:(1)Allobjectsofaclassofthingsareexaminedinthepremise.(2)Theassertionsmadeinthepremiseforeachobjectofthistypeofthingaretrue.
Function
Completelyinductivereasoninghastwofunctions:
(1)Cognitivefunction.Completelyinductivereasoningisbasedonthefactthateachobjectofacertaintypeofthingshasacertainattribute,anditisconcludedthatthistypeofthingsallhavethisattribute,whichmakespeople'sunderstandingrisefromindividualtogeneral.Forexample,theaboveconclusionbasedonthe"continentsontheearth"categoryofthingsthateachobjecthastheattribute"hasmineraldeposits",andtheconclusionthat"allcontinentsontheearthhavemineraldeposits"reflectstheunderstandingofcompleteinductivereasoning.effect.
(2)Demonstrationfunction.Becausetheconnectionbetweenthepremiseandtheconclusionofcompleteinductivereasoningisinevitable,itisoftenusedasapowerfulmethodofargumentation.Forexample,forthetopic"Thesyllogismoftwospecialpremisescannotinferaconclusion",itcanbearguedlikethis:thepremiseisthatthesyllogismofIIcannotinferaconclusion,thepremiseisthatthesyllogismofOOcannotinferaconclusion,andthepremiseisthatthesyllogismofIO(OI)isinferred.Noconclusion,thepremiseisthesyllogismofII,thepremiseisthesyllogismofOO,andthepremiseisthatthesyllogismofIO(OI)isalltheobjectsofthetwosyllogismsofspecialpremises.Therefore,thesyllogismoftwospecialpremisescannotinferaconclusion.
Completelyinductivereasoningusuallyappliestoasmallnumberofthings.Whenthenumberofthingstobeinvestigatedisextremelylarge,eveninfinite,completeinductivereasoningisnotapplicable,andanotherformofinductivereasoningneedstobeused,thatis,incompleteinductivereasoning.
Incompletemethod
Concept
Incompleteinductivereasoningisbasedonthefactthatsomeobjectsofacertainkindofthingshavecertainattributes,andthusitisconcludedthatallkindsofthingshavecertainattributes.Theconclusionofthisattribute.Incompleteinductivereasoningincludessimpleenumerationinductivereasoningandscientificinductivereasoning.
Simpleinductivereasoning
Inaclassofthings,someoftheobjectsthathavebeenobservedhavecertainattributes,andnocounterexampleshavebeenencountered,soitcanbeconcludedthatthisclassofthingshasTheconclusionofthiskindofattributeissimpleenumerationandinductivereasoning.Forexample,the"GoldbachConjecture",knownasthe"jewelinthecrownofmathematics,"wasputforwardusingsimpleenumerationandinductivereasoning.Morethan200yearsago,GermanmathematicianBrotherDebachdiscoveredthatsomeoddnumbersareequaltothesumofthreeprimenumbers.Forexample:
17=3+3+11
41=11+13+17
77=7+17+53
461=5+7+449
Goldbachdidnotlistalltheoddnumbers(infact,itisimpossible),onlyafewexamplesConjecture:Alloddnumbersgreaterthan5canbedecomposedintothesumofthreeprimenumbers.HetoldthisconjecturetoMathematicianEuler.Eulerconfirmedhisconjectureandaddedaconjecture:Evennumbersgreaterthan4canbedecomposedintothesumoftwoprimenumbers.Forexample:
10=5+5
14=7+7
18=7+11
462=5+457
Thepreviouspropositioncanbeprovedfromthisproposition,andthesetwopropositionswerelatercollectivelycalledthe"GoldbachConjecture".
Manyfolkproverbs,suchas"RuixueZhaofengYear","Thefoundationismoistandrain,themoonishazyandthewind","Birdsflylow,putontheclothes",etc.,arebasedonrepeatedmanytimesinlife.Examplesaresummarizedbysimpleenumerationandinductivereasoning.
Thelogicalformofsimpleenumerationinductivereasoningisasfollows:
S1isP
S2isP
……
SnisP
S1,S2,...,SnispartoftheSclass,andnoSisnotP
So,allSis(orisnot)P
Theconclusionofsimpleenumerationandinductivereasoningisprobable,becauseitsconclusionisbeyondthescopeofknowledgedeterminedbythepremise.MathematicianHuaLuogenggaveagooddescriptionoftheprobabilityofsimpleenumerationinductivereasoninginthebook"MathematicalInduction":
"ThefirstoneoutofabagisredGlassballs,thesecondoneisredglassballs,evenwhenthethird,fourth,andfifthareredglassballs,weimmediatelyguess:'Arealltheballsinthebagredglassballs?'However,oncewetouchedoutawhiteglassball,thisconjecturefailed.Atthistime,wewillhaveanotherconjecture:'Iseverythinginthebagallglassballs?'Whenwetouchedoutawoodenballonce,Thisconjecturefailedagain.Atthattime,wewillhaveathirdconjecture:"Isthebaginthebagallballs?"Thisconjectureiscorrect,wemustcontinuetotest,tofindoutallthecontentsinthebagInordertoseeadifference."
Toimprovethereliabilityofsimpleenumerationinductivereasoning,wemustpayattentiontothefollowingtworequirements:(1)Thenumberofenumerationsmustbelargeenough,andthescopeofinvestigationmustbewideenough.(2)Checkwhethertherearecounterexamples.Usually,simpleenumerationandinductivereasoningthatdoesnotpayattentiontotheabovetworequirementsandthereforehastoofewsamplesandtheconclusionisobviouslyfalseiscalled"tobepartial"or"togeneralizelightly".
LuXunwrotein"ThePrefaceofUchiyamaFinishingWorks":"AtravelerwalkedintothestudyroomofarichofficialinXiayeandsawmanyexpensiveinkstones.ShisaidthatChinaisa'gentlecountry';whenanobservercomestoShanghai,buysseveralobscenebooksandpictures,andthenlooksforstrangethingstoobserve,hecallsChinaa'pornographiccountry'."Inthisarticle,LuXunfurtherrevealedthatbecauseoftheinsufficientnumberofenumerationsortheinsufficientscopeofinvestigation,suchpeopledidnotpayattentiontoinvestigatingwhethertherewerecounterexamples,sothattheywere"partiallycomprehensive"or"indiscreetlygeneralized"andinevitableintheend.Thedilemmatofallinto:"Ifyougotoapoorliterati’shomeorapartment,youdon’tcareaboutyourstudy,eventheinkstonecostsonlytwodimes.Onceyouseesuchathing,thepreviousconclusionwillnotpass.Soobserversarealittleembarrassed."
Simpleenumerationinductivereasoningisthesimplestmethodofinductivereasoning.However,despitethis,itssignificancecannotbeignored.
(1)Simpleenumerationandinductivereasoningcanhelpdiscovery.Whenasufficientbasisforthegeneralizationcannotbefound,butthereisconsiderablematerial,itisnecessarytousesimpleenumerationandinductivereasoningtomakeapreliminarygeneralizationandcomeupwithaprobableconclusionasastartingpointforfurtherresearch.Therefore,simpleenumerationandinductivereasoningareoftenusedwhenforminghypotheses.Forexample,inthediscoveryprocessofBoyle'sLaw,simpleenumerationandinductivereasoningplayedacertainrole.Boylesummedupthelawthat"undercertainconditions,thevolumeofagasisinverselyproportionaltothepressureitreceives"frommanyexperimentalfactshehasmastered.
(2)Simpleenumerationandinductivereasoningcanalsobeusedasamethodofargumentationandplayacertainroleintheprocessofargumentation.Forexample,HuShihadaconversationinhislateryears:"Anyonewhoisverysuccessfulissomeonewhoisextremelysmartandwillingtododumbskills.NotonlyinChina,butalsointheWest.LikeConfucius,hesaid,"Idon’teatalldayandsleepallnight,thinking,it’suseless,it’sbettertolearn.ThisistheworkofConfuciusinlearning.ChinesemathematicianandlinguistZhouHaizhongb>FormanyyearsofresearchonMersenneprimenumbers,heusedtheconnectionobservationmethodandtheincompleteinductionmethodtofirstgivethepreciseexpressionofthemersenneprimenumberdistributionin1992,thusrevealingtheimportantlawsofMersenneprimenumbers.,Whichprovidesconvenienceforpeopletoexplorethisprimenumber.Laterthisscientificresearchresultwascalled"Zhou’sguess"internationally.
Scientificinductivereasoning
ScientificinductivereasoningisbasedonacertainTheanalysisofthecausalrelationshipbetweensomeobjectsinaclassofthingsandacertainattribute,andthereasoningthatthisclassofthingshasthatattribute.Forexample:
Thevolumeofgoldexpandsafterheating;
SilverisheatedAfterthevolumeexpansion;
Thevolumeexpandsafterthecopperisheated;
Thevolumeexpandsaftertheironisheated;
Becausethemetalisheated,themolecularcohesionweakensandthemolecularmotionaccelerates,Thedistancebetweenmoleculesincreases,whichleadstoexpansion,whilegold,silver,copper,andironareallmetals;
So,allmetalsexpandinvolumeafterbeingheated.
Thepremisenotonlyexaminesthatsomeobjectsofaclassofthingshavecertainattributes,butalsofurtherpointsoutthecausalconnectionbetweenobjectsandattributes,andconcludesfromthis.Thisisscientificinductivereasoning.
ScientificinductivereasoningTheformisasfollows:
S1isP
S2isP
……
SnisP
S1,S2,...,SnarepartoftheobjectsofclassS,andthereisnoSi(1≤i≤n)whichisnotP;andscientificresearchshowsthatthereisacausallinkbetweenSandP
So,allSisallP
Comparedwithsimpleenumerationinductivereasoning,scientificinductivereasoninghasthesameanddifferentpoints.
Thecommonpointisthattheyallbelongtoincompleteinductivereasoning.Thepremisesareonlypartoftheobjectsofoneclassofthings,andtheconclusionsarealltheconclusionsofthewholegroupofthings,andthescopeoftheknowledgeoftheconclusionsexceedsthepremise.
Thedifferenceis:(1)Thereasoningisdifferent.Simpleenumerationinductivereasoningisbasedonlyonthefactthatsomeoftheobjectsthathavebeenobservedhavecertainattributesandhavenotencounteredanycounterexamples.Scientificinductivereasoningdoesnotstopatrepeatingtheexperienceofthings,butconductsin-depthscientificanalysisandgraspsConclusionsaremadeonthebasisofthecausalconnectionbetweenobjectsandattributes.
(2)Thenumberofpremiseshasdifferentmeaningsforthetwo.Forsimpleenumerationandinductivereasoning,themoreobjectsareexaminedinpremises,Thewiderthescope,themorereliabletheconclusion.Forscientificinductivereasoning,thenumberofpremisesdoesnothaveadecisivesignificance,aslongasthecauseandeffectbetweentheobjectandtheattributeisfullyunderstoodConnection,evenifthenumberofpremisesissmall,evenifthereareonlyoneortwotypicalcases,reliableconclusionscanbedrawn.AsEngelssaid,ahundredthousandsteamengineisnobetterthanasteamenginetoexplaintheconversionofheatenergyintomechanicalenergy.
(3)Thereliabilityoftheconclusionisdifferent.Althoughtheconnectionbetweenthepremisesandconclusionsofthetwoisprobable,theintensityofinductionisnotnecessarilyequalto1.However,scientificinductivereasoningexaminesthecausalconnectionbetweenobjectsandattributes.Therefore,theinductivestrengthofscientificinductivereasoningisstrongerthanthatofsimpleenumerationinductivereasoning.Thatistosay,scientificinductivereasoningiscomparedwithsimpleenumerationinductivereasoning.Theconclusionishighlyreliable.
Scientificinductivereasoningadvocatesahabitofthinkinginthefaceofknowledgeandconclusions.Thishabitisespeciallyimportantintheageofadvancedinformation.Thinkaboutit,ourmediaoftendisseminatessomecontradictory"scientificknowledge"tous,thisisnotdifficulttounderstand.
Forexample,themediasometimessaythatonehundredstepsafteramealisgood;sometimestheysaythatonehundredstepsafteramealisnotgood.Foranotherexample,sometimesitissaidthatovernightteacannotbedrunk,whichisharmfultohealth;sometimesitissaidthatstudieshaveshownthatovernightteacanbedrunkjustlikenon-overnighttea.Andsoon,peoplearesimplyoverwhelmed.Themainfeatureofscientificinductivereasoningistoinvestigatethecausalconnectionbetweentheobjectandtheattribute,whichhelpstoguidepeopletoexploretheessenceofthings,discoverthelawsofthings,andmorereliablypromoteperceptualknowledgetorationalknowledge.
ProbabilisticReasoning
M.Kleinwrotein"MathematicsinWesternCulture":"Needlesstosayaboutourfuture,evenonehourfromnow,Andthereisnothingforsure.Afteraminute,thegroundunderourfeetmaycrack.However,claimingthispossibilitycannotscareus,becauseweknowthattheprobabilityofthissituationisextremelysmall.
Inotherwords,itistheprobabilityofwhetheraneventoccursornot,whichdeterminesourattitudesandactionstowardstheevent."ThephenomenonthatmayormaynotappearundercertainconditionsiswhatwecallItisarandomeventoraccidentalevent,suchasdrawingakingofheartsfromadeckofbridgecards.Infact,whenweobservealargenumberofrandomeventsofthesamekind,wewillfindthatthereisacertainregularityamongthem.
Probabilityisthequantitativedescriptionofthelawpresentedbyalargenumberofrandomevents,usuallyexpressedbyP(A).Usingprobabilisticreasoning,wecanlearnhowlikelyaneventis,orhowlikelyitistohappen.Inthissense,itcanbesaidthatprobabilisticreasoningistheinferenceaboutchance.
Probabilityvalue
Indailylife,weareonlysatisfiedwithestimatingwhethertheprobabilityofaneventishighorlow.However,thisestimateistoobroadtomeettheneedsofmanyissuessuchasindustry,economics,insurance,medicalcare,sociology,psychology,andsoon.Becauseintheabovesituation,theexactprobabilityvaluemustbeknown.Toachievethisgoal,youneedhelpfrommathematics.Relyingontheprobabilityvaluecalculatedbymathematicscanwereliablyguideouractions.
Generally,thedefinitionofcalculatingtheprobabilityvalueis:iftherearenkindsofequalpossibilities,andthesituationthatisconducivetotheoccurrenceofaneventism,thentheprobabilityoftheoccurrenceoftheeventism/n,theprobabilityofnothappeningis(nm)/n.Underthisdefinition,iftheeventisimpossible,theprobabilityoftheeventis0/n,whichis0;iftheeventiscompletelycertain,theprobabilityisn/n,whichis1.
Therefore,theprobabilityvaluevariesfrom0to1,thatis,fromimpossibilitytocertainty.Theso-calledequalpossibilitymeansthatthelikelihoodofoccurrenceisthesame.Forexample,adicehas6sides.Ifthereisnofactorintheshapeofthediceorinthewayofthrowingthedice,thereisnofactorinfavoroftheappearanceofacertainside,thentheprobabilityoftheappearanceof6sidesofthediceisthesame,thatis,thedicehas6types,etc.possibility.
Accordingtothisdefinitionofcalculatingtheprobabilityvalue,theprobabilityofselectingacard"A"fromadeckof52ordinaryunsoapedplayingcardsis4/52,or1/13.Becausethereare52suchpossibilities,4ofthemareadvantageous.However,ifallpossibilitiesarenotequallypossible,thenthisdefinitionofcalculatedprobabilityvaluedoesnotapply.Forexample,thereareonlytwopossibilitiesforapersontocrossthestreet:eithersafelyornot.However,itcannotbeconcludedfromthisthattheprobabilityofapersoncrossingthestreetsafelyis1/2,becausethetwopossibilitiesof"passingsafely"and"passingwithoutsafety"arenotequallypossible.
Itshouldbenotedthatprobabilitytellsuswhathappensinalargeselection.Forexample,theprobabilityofchoosingan"A"from52decksofcardsis1/13.Thisdoesnotmeanthatifapersontakes13timesfromthisdeckofcards,hewilldefinitelychoosean"A".".Hemayhavefetched30or40times,butdidnotgetan"A".However,themoretimeshetakes,theratioofthenumberofAcestothetotalnumberofcardswillbecloseto1/13.Inaddition,thisdoesnotmeanthatifapersontakesan"A",forexample,ithappenstobethefirsttime,theprobabilityoftakingan"A"nexttimemustbelessthan1/13.Theprobabilitywillstillbethesame,whichis1/13,evenifthe3"A"saretakenoutinsuccession.Becauseadeckofcardshasneithermemorynorconsciousness,sowhathashappenedwillnotaffectthefuture.
